A generalization of Kantorovich operators and a shapepreserving property of Bernstein operators

Radu Paltanea

Authors

Radu Paltanea Transilvania University of Brasov, Romania

Keywords:

Kantorovich type operators, Bernstein operators, shape-preserving property

Abstract

We construct a generalization of the Kantorovich operators, depending on a parameter b ≥ 0 and we prove that if a function f ∈ C¹[0, 1] with f (0) = 0, satisfies the differential inequality f ' + bf ≥ 0, then functions B_n(f ), n ∈ 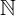$\mathbb{N}$ satisfy the same inequality, where B_n is the Bernstein operator.